Free Worksheets Maths: four rules

Standard method of addition of 4-digits (2)

Here is another maths worksheet of addition of two 4-digit numbers. To be successful with these children need to have a very good knowledge of number bonds up to ten eg know that 6 + 5 is 11. They must also be confident with place value, understanding that each column of numbers needs to be vertically in a line.

A full explanation of this method can be found on the first of the adding two 4-digit numbers worksheet.

This page and other similar standard written addition worksheets, can be found in our Four Rules, Addition section.

Standard addition of 4-digits (pg 2)

Standard written addition of 4-digits

Addition of two 4-digit numbers is usually done on paper, using the following standard method:

The method is to add the units first, put the units in the answer, and ‘carry’ the ten into the tens column. Then add the tens and continue in the same way into the hundreds and finally the thousands.

A clearer explanation is available on the first page of the worksheets, together with a page of questions, but briefly:

Looking at 5687 + 2546 the steps are:

Step 1: add the units

7 + 6 = 13

Put the 3 in the units below the question.
Then place the one ten below the answer in the tens column.

Step 2: add the tens
8 (tens) + 4 (tens) + 1 (ten) = 13 (tens)
Place the 3 (tens) in the tens column and the 1(hundred) in the hundreds column below the answer.

Step 3: add the hundreds
6 (hundreds) + 5 (hundreds) + 1 (hundred) = 12 (hundreds)
Place the 2 (hundreds) in the hundreds column and the 1(thousand) in the thousands column below the answer.

Note: there may not always be tens, hundreds or thousands to carry.

Step 4: add the thousands
5 (thousands) + 2 (thousands) + 1 (thousand) = 8 (thousands)
Place the 8 (thousands) in the thousands column.
Answer: 8233

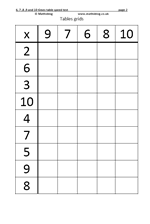
Standard addition of 4-digits (pg 1)

Subtraction of money

This is another in our series of worksheets covering the four rules. Children should be competent with the standard written method of subtraction before moving onto subtracting decimals. Money is an excellent way to show how decimals work and it is important to ensure that there are two numbers after the decimal point. Another key point, of course, is to make sure the columns are correctly in line and that a decimal point is included in the answer. Make sure that questions 9 to 15 are written out in the correct format: vertically rather than horizontally.

Standard money subtraction (p1)

Maths worksheet: Add decimals mentally (1)

At first glance you might reach for a pencil to do a sum such as 2.5 + 4.7. However, the Primary Framework for Maths suggests that children should be competent in adding two 2-digit numbers in their heads, and there is no reason why, with a little practice this can’t include adding two decimals. The most common approach is almost identical to adding tens and units.

Start by adding the largest digits, in this case the units: 2 + 4 = 6.

Then add the larger of the two tenths digits, which is 0.7, making 6.7.

Finally add on the 0.5, by counting on if necessary, making 7.2. Easy!

Add two decimals mentally (1)

There are, of course other approaches, such as adding 2 to the 4.7 to make 6.7, then adding the 0.5, which are equally good. It often depends on the numbers involved and any method which is quick and accurate is fine!

Maths worksheet: Long division p2

The second in our series of long division pages. These are very hard for primary school children and should not be attempted until they have a good knowledge of tables, multiplying and subtraction.

Example: 789 divided by 36.

First carry out an estimate of the answer. I think 789 divided by 36 is about 20.
Then proceed using these steps:
1. How many 36s in 78?
2. 2 x 36 is 72. 3 x 36 is 108 which is too many, so it must be 2.
3. Put the 2 in the tens column above the answer.
4. Place the 72 below the 78 and subtract.
5. 78 – 72 is 6.
6. ‘Bring down’ the 9 to make 69.
7. How many 36s in 69.
8. By trial and improvement and some rough work multiplying 36 by my estimated numbers I find that 36 x 1 = 36.
9. Place the 36 under the 69 and subtract.
10. The remainder (33) must be less than the original number you are dividing by.

Long division (pg 2)

Learning tables: 6x, 7x, 8x, 9x and 10x tables pg 2

This is the second page for tables 6 to 10. The idea is to fill in the grid as quickly as possible. The tens are easy, although never suggest that ‘add a naught’ will give the correct answer! The nines are interesting in that the digits have a digital root of 9 when added (1 + 8 = 9, 2 + 7 = 9 etc). The six, seven and eight times tables are probably the hardest for most children to learn.
When completing the grid various tactics can be used if the tables have not been thoroughly learned. For example, 6 x 9 is just 6 less than 6 x 10; 7 x 9 is 7 less than 7 x 10 etc.
Also if they know that 6 x 7 = 42 then use this to answer 7 x 6, which will also be 42.
However, the ultimate aim is to know tables off by heart.

6, 7, 8, 9 and 10 x tables pg 2

Maths worksheet: Long division

Perhaps the hardest thing that children are asked to do in primary school is long division – and many children never master it, not even later in life. The main reason it is so difficult is that involves a number of stages, a good knowledge of tables and multiplication as well as subtraction. Looking at a problem such as 867 divided by 34, there are at least ten distinctive stages to go through:

34)867
First carry out an estimate of the answer. I think 867 divided by 34 is over 20 but under 30.

Then proceed using these steps:
1. How many 34s in 86?
2. 2 x 34 is 68. 3 x 34 is 102 which is too many, so it must be 2.
3. Put the 2 in the tens column above the answer.
4. Place the 68 below the 86 and subtract.
5. 86 – 68 is 18.
6. ‘Bring down’ the 7 to make 187.
7. How many 34s in 187.
8. By trial and improvement and some rough work multiplying 34 by my estimated numbers I find that 34 x 5 = 170.
9. Place the 170 under the 187 and subtract.
10. The remainder must be less than the original number you are dividing by.

Long division (pg 1)

Maths worksheet: Standard subtraction of 3-digits

Here is a basic set of subtraction questions using 3-digit numbers. They should be tackled in the standard method as described in earlier 2-digit subtraction worksheets. Notice that there are no zeros in the tens column as this can lead to particular problems and needs a set of questions all of its own.

The last three questions have been set out in a horizontal form. To answer them they should be rewritten in the standard way. Look out for mistakes where decomposition was needed but where the subtraction has been reversed eg 3 – 7 has been done as 7 – 3.

If these questions are answered correctly and in the correct method then it can be said that mastery of the subtraction method is well on its way!

Standard subtraction of 3 digits (p1)

Mental arithmetic: Dividing by 4

A nifty way of dividing a number by 4 is to halve the number and then halve again. This method is much easier with even numbers and, of course, whole multiples of 4, but can be used for most 2-digit numbers – just the remainders become tricky. All these questions are multiples of 4 so should be relatively straightforward. Knowing these ‘tricks’ can give children a lot of confidence and can also be a useful way of checking answers which have been calculated on paper, or even on a calculator, to ensure that the answer is sensible.

Divide by 4 mentally

Multiply by 9 mentally (1)

You can multiply most 2-digit numbers by 9 in your head. The most efficient way is to multiply the number by ten and then subtract the number. For example:

45 x 9

First of all multiply 45 by 10 which is 450.

Then subtract 45 from 450 which is 405.

This maths worksheet shows how to do this and then gives some practice questions – but you do have to be pretty good at subtraction to use this method.

Multiply by 9 mentally (1)