The big question: how do you put fractions in order of size? It is easy to compare fractions if the bottom number (the denominator) is the same for each fraction. 3/12 is smaller than 5/12 etc. But if the denominators are different it becomes more tricky.
The big question: how do you put fractions in order of size? It is easy to compare fractions if the bottom number (the denominator) is the same for each fraction. 3/12 is smaller than 5/12 etc. But if the denominators are different it becomes more tricky.
There are several ways to do this (eg treat each fraction as a division sum and use a calculator to work out the division answers and then order them) but the established method is to change each fraction so that they all have the same denominator.
Warning! If children are going to be successful with this there are several things that they already need to be confident with, including knowing tables and being able to recognise multiples of a number. They also need to know that multiplying the numerator (top) and denominator (bottom) of a fraction by the same number will not change the fractions size – equivalent fractions again! Without this knowledge they will be doomed and confused!!
Let’s take 3/4 and 2/3 as a simple example. To be able to directly compare them the denominators of both fractions need to be the same. Both fractions can be shown in twelfths. (If you don’t understand why I have chosen twelfths then have a look at some of the equivalent fraction pages on the site.)
3/4 can be changed by multiplying 4 by 3 and then 3 by 3 to give 9/12.
2/3 can be changed by multiplying 3 by 4 and 2 by 4 to give 8/12
From this it can be sen that 2/3 or 8/12 is smaller than 3/4 or 9/12.
There is a more detailed explanation on ordering fractions on the maths worksheet pages.
Ordering fractions p1
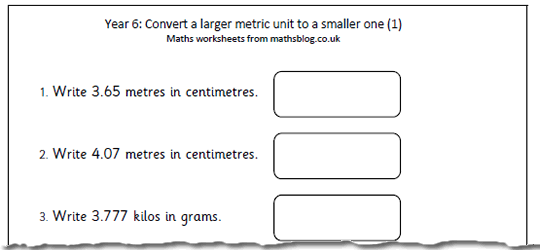
 This is about as hard as it gets for converting larger metric units to smaller ones. The larger units all include decimals and a good knowledge of multiplying by 10, 100 or 1000 is needed.
This is about as hard as it gets for converting larger metric units to smaller ones. The larger units all include decimals and a good knowledge of multiplying by 10, 100 or 1000 is needed.